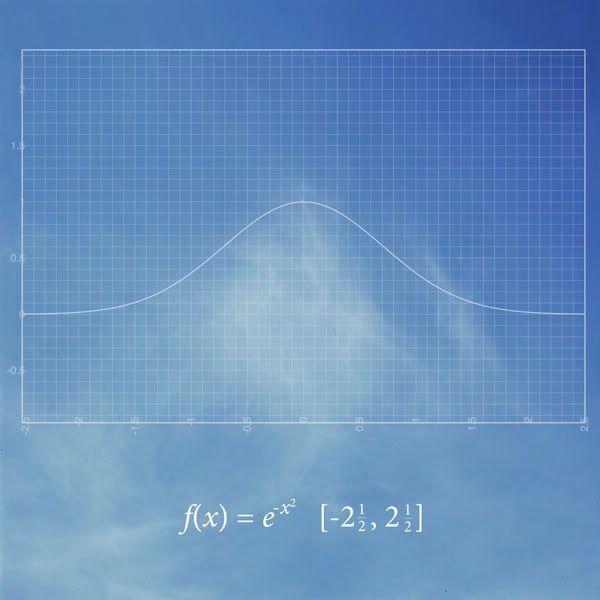
Ah! It's our old friend, the Gaussian distribution in the sky. This function is in a family with the Normal distribution (commonly known as the bell curve) and useful for thinking about standard deviation.

Now, this, to a physicist accustomed to time series analysis is a Bartlett window. See, if you need to break something into a summed series of sinusoids, it is important to break it into equal bits (windows of data) and taper the ends. So, you multiply the bits by something which is low at either end and high in the middle. This is one of those tools in my signal processing toolbox.

This is a hyperbolic paraboloid, or a saddle surface, something like z=x2−y2. It has negative Gaussian curvature, which is a fancy way of saying that if you draw a triangle on a saddle the sum of the angles will be less than 180o (the sum of the angles if the triangle is drawn on a flat sheet of paper). Physicists know that gravitation is geometry and have to think about this sort of mathematical creature when considering general relativity because mass deforms the curvature of spacetime itself.

This function isn't in my mathematical zoo. I don't know its purpose, though I can see how it works. Pretty, isn't it? The fact that I think of functions in terms of "purpose" as opposed to "beauty" distinguishes me as a physicist, rather than a mathematician. But, I really appreciate seeing these Found Functions.
{via today and tomorrow}






No comments:
Post a Comment